The derivative of an inverse function calculator is designed to compute the derivative of the inverse of a given function. This is particularly useful in advanced calculus where finding the slope of tangent lines to inverse functions manually can be arduous and time-consuming. By automating these calculations, the calculator aids in accurate and swift problem-solving.
Formula of Derivative of Inverse Function Calculator
Understanding the formula used by the calculator is key to leveraging its capabilities:
The formula for the derivative of an inverse function:
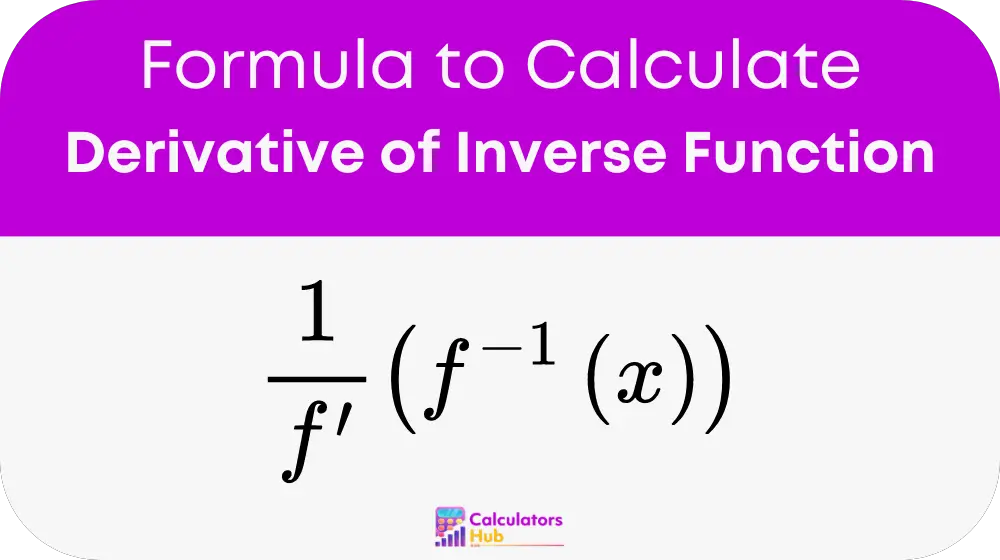
This formula signifies that the derivative of an inverse function at a point is the reciprocal of the derivative of the original function at the corresponding point. Here, f−1(x) represents the inverse function, while f′(x) denotes the derivative of the original function with respect to x.
Table of General Terms and Applications
To aid in the comprehension and application of this calculator, below is a table outlining key terms and their significance:
| Term | Definition | Application Example |
|---|---|---|
| Inverse Function | A function that reverses another function | Reversing y=2x to x=y/2 |
| Derivative | Measures how a function changes as its input changes | Determining the slope at a point |
| Reciprocal Derivative | The reciprocal of the derivative of a function | Used in the formula for inverse derivatives |
Example of Derivative of Inverse Function Calculator
Let’s consider the function f(x)=log(x), whose inverse is f−1(x)=ex. Applying the calculator to find the derivative of the inverse at x=1 involves the following steps:
- Identify f′(x) of the original function, which is 1/x.
- Plug x=1 into the inverse function, giving e1.
- Evaluate f′(e) and apply the formula to find 1 / f′(e).
The calculated result provides the derivative at the specified point, enhancing understanding and facilitating further analysis.
Most Common FAQs
The output represents the slope of the tangent line at the given point on the inverse function, which is crucial for understanding the function’s behavior around that point.
Ensure the function and its inverse are correctly identified and that the derivative is accurately calculated before applying the formula.
It serves as an excellent teaching aid for demonstrating the practical applications of derivatives in real-world scenarios, making abstract concepts more tangible.