The Coterminal Angle Calculator is a tool that helps users find coterminal angles, which are angles that share the same terminal side on the unit circle, meaning they represent the same position despite having different values. In other words, coterminal angles differ by multiples of 360° (or 2π radians) and can be used interchangeably in various mathematical and scientific calculations.
Coterminal angles are especially useful in trigonometry and geometry, where simplifying angles to a specific range (typically between 0° and 360° or 0 and 2π radians) is often required for easier calculations. This calculator allows users to determine these angles quickly, whether working with degrees or radians.
Formula of Coterminal Angle Calculator
The formula to calculate coterminal angles is as follows:
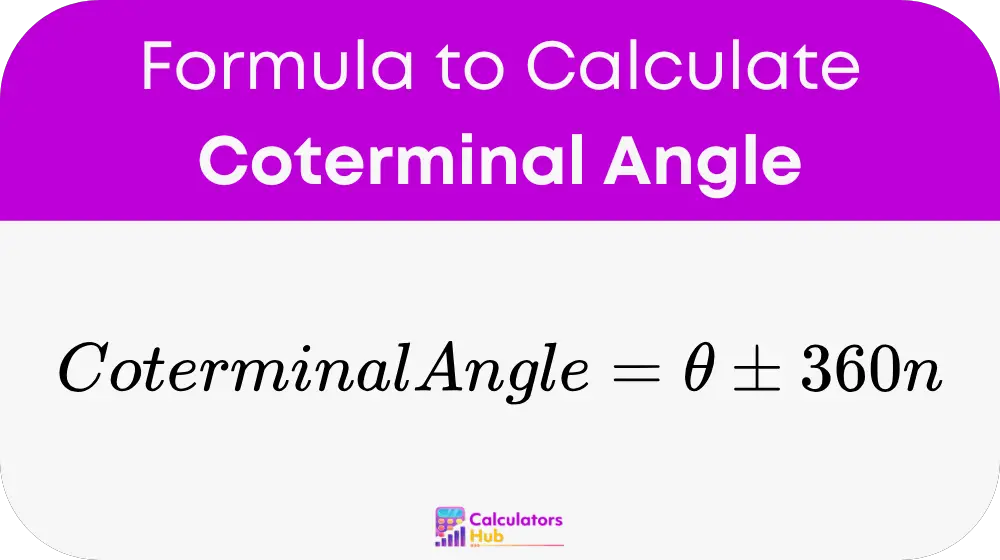
Where:
- θ is the original angle, expressed in degrees or radians.
- n is an integer (positive or negative), representing how many full rotations (360° or 2π radians) to add or subtract from the original angle to find a coterminal angle.
By applying this formula, users can generate coterminal angles within the desired range, such as finding an angle between 0° and 360° (for degrees) or 0 and 2π radians (for radians).
General Terms
Here are some common terms related to coterminal angles that people often search for and may find helpful when using the Coterminal Angle Calculator:
| Term | Description |
|---|---|
| Coterminal Angles | Angles that share the same terminal side on the unit circle but differ by multiples of 360° or 2π radians. |
| θ (Theta) | A variable commonly used to represent an angle in mathematics, particularly in trigonometry. |
| 360° (Degrees) | A full rotation of a circle, commonly used to measure angles in degrees. |
| 2π (Radians) | A full rotation of a circle in radians, equivalent to 360°. |
| Unit Circle | A circle of radius 1 centered at the origin of the coordinate plane, used to define trigonometric functions. |
| Positive Integer (n) | A whole number used in the formula to indicate the number of full rotations to add or subtract to an angle. |
| Negative Integer (n) | A negative whole number indicating that rotations are to be subtracted from the angle. |
| Principal Angle | The smallest positive angle that is coterminal with a given angle, typically between 0° and 360° (or 0 to 2π radians). |
| Trigonometric Functions | Mathematical functions (sine, cosine, tangent, etc.) that relate the angles of a triangle to the lengths of its sides. |
This table helps users understand the terminology related to coterminal angles, which is crucial for applying the formula and interpreting the results.
Example of Coterminal Angle Calculator
Let’s walk through an example to see how the Coterminal Angle Calculator works.
Suppose you are given the angle θ = 450° and want to find its coterminal angle within the range of 0° to 360°.
- Start with the formula: Coterminal Angle = θ ± 360n
- Substitute the given angle: Coterminal Angle = 450° ± 360n
- Now, subtract 360° (since we are looking for a smaller coterminal angle within the 0°-360° range):
- 450° – 360° = 90°
Thus, 90° is a coterminal angle of 450°, meaning they share the same terminal side.
Let’s look at another example with a negative angle. If θ = -30°, you can find a coterminal angle within the 0° to 360° range:
- Use the same formula: Coterminal Angle = θ ± 360n
- Substitute the given angle: Coterminal Angle = -30° ± 360n
- Now, add 360°:
- -30° + 360° = 330°
So, 330° is a coterminal angle of -30°.
These examples demonstrate how to calculate coterminal angles for both positive and negative original angles.
Most Common FAQs
To calculate coterminal angles, use the formula Coterminal Angle = θ ± 360n, where θ is the given angle and n is an integer. By adding or subtracting multiples of 360° (or 2π radians), you can find angles that share the same terminal side on the unit circle.
Coterminal angles are widely used in trigonometry, physics, engineering, and computer graphics. They simplify calculations by reducing angles to a standard range, often between 0° and 360° (or 0 and 2π radians). For example, in navigation and signal processing, coterminal angles help describe periodic functions and rotations.
The principal angle is the smallest positive coterminal angle, usually found between 0° and 360° (or 0 and 2π radians). It’s often the simplest angle to work with when solving trigonometric problems or simplifying expressions.