A Complex to Polar Calculator is a tool designed to convert complex numbers from their rectangular (a + bi) form to polar coordinates (r, theta). This transformation is invaluable in fields like electrical engineering and physics, where complex numbers are frequently used to represent waveforms, impedances, and more. By inputting the real (a) and imaginary (b) parts of a complex number, the calculator provides the magnitude (r) and argument (theta) of the number in polar form, offering a different perspective and simplification of complex calculations.
Formula of Complex to Polar Calculator
To understand the operation of a Complex to Polar Calculator, it’s essential to familiarize oneself with the formulas it uses:
Magnitude (r):
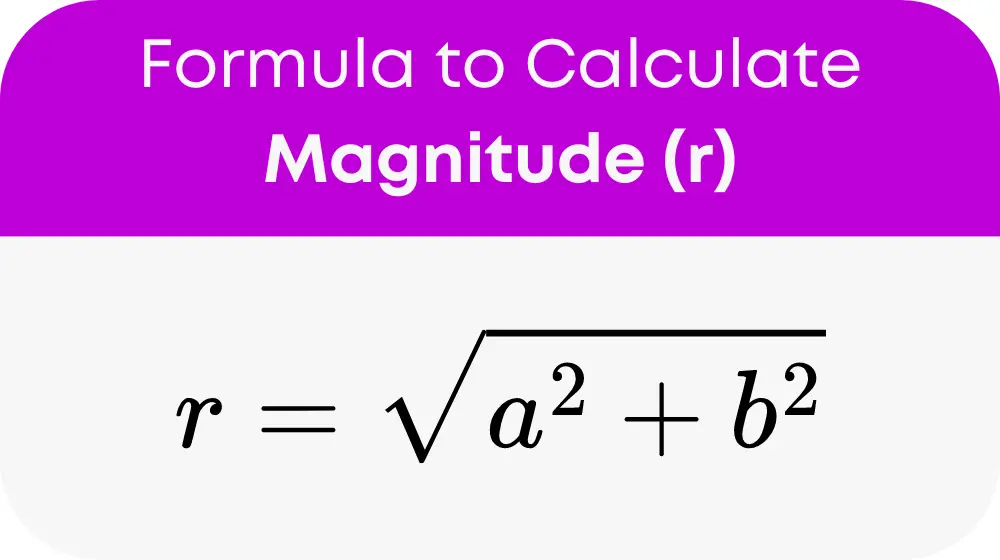
Here, r represents the modulus or absolute value of the complex number. It signifies the distance from the origin (0, 0) to the point representing the complex number on the complex plane.
Argument (theta):
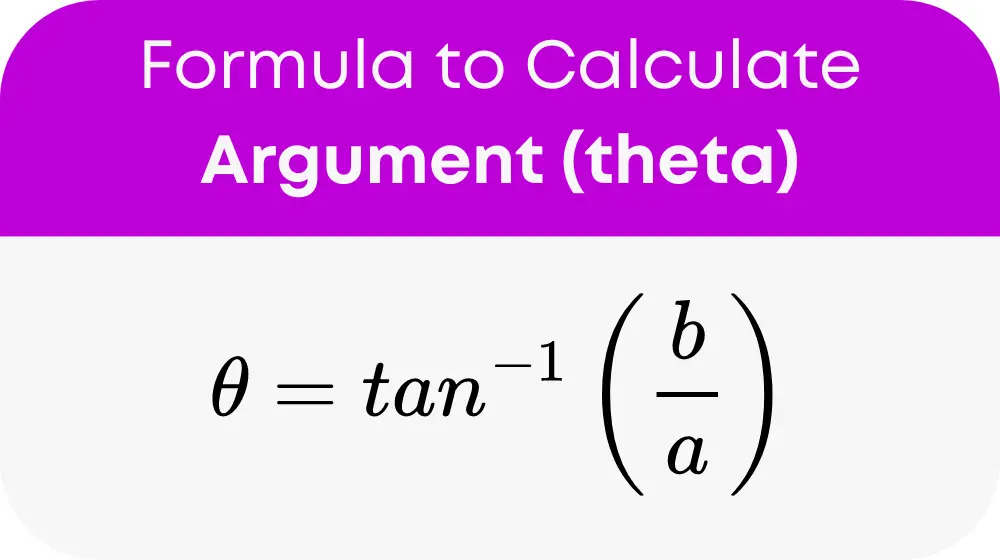
Note:
- The arctangent function (atan) has multiple possible outputs depending on the quadrant where the complex number lies. Some calculators include a special function (atan2) that considers both the signs of a and b to provide the correct angle in all quadrants.
- The angle theta can also be expressed in degrees using the conversion factor: degrees = radians × 180 / pi.
General Terms Table
To aid understanding and practical use, below is a table outlining common terms related to complex numbers and their conversions:
| Term | Definition | Example |
|---|---|---|
| Complex Number | A number of the form a + bi | 3 + 4i |
| Real Part | The a in a + bi | 3 |
| Imaginary Part | The b in a + bi, where i = sqrt(-1) | 4 |
| Magnitude | Distance from the origin to the point (a, b) on the complex plane | 5 for 3 + 4i |
| Argument | The angle formed with the positive real axis | 53.13 degrees for 3 + 4i |
This table serves as a quick reference to understand and use the Complex to Polar Calculator more effectively without needing to perform each calculation manually.
Example of Complex to Polar Calculator
Consider the complex number 3 + 4i. Using the formulas:
- Magnitude: r = sqrt(3^2 + 4^2) = 5
- Argument: theta = atan(4/3) = 53.13 degrees
This example demonstrates how the calculator simplifies the conversion process, providing clear, understandable results.
Most Common FAQs
Rectangular form represents a complex number as a + bi, focusing on the horizontal (real) and vertical (imaginary) components. Polar form, on the other hand, represents the number in terms of its magnitude and angle, highlighting its directional qualities.
Enter the real and imaginary parts of the complex number into the calculator. It will automatically compute and display the magnitude and argument in polar form.
Polar form simplifies multiplication, division, and finding powers and roots of complex numbers, making it highly beneficial for advanced mathematical, engineering, and physics applications.