The Cardioid Area Calculator is an invaluable tool designed to compute the area enclosed by a cardioid curve efficiently. This tool is essential for students delving into advanced mathematics, educators crafting illustrative examples, and professionals involved in design and analysis where precise area calculations are necessary.
Formula of Cardioid Area Calculator
The area of a cardioid is computed using the formula:
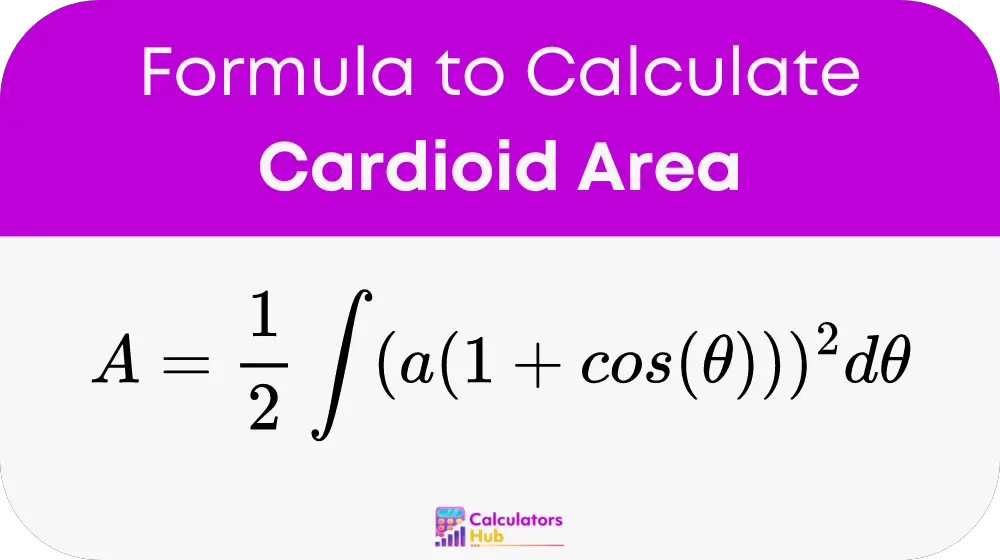
Here’s a breakdown of the formula components:
- A: Represents the area enclosed by the cardioid.
- a: A parameter that defines the size of the cardioid. It is the distance from a fixed point to a point on the curve when theta = 0.
- theta: The angle variable in the polar coordinate system.
- Integral: Symbolizes integration, indicating a sum over a continuous range.
Table of Pre-Calculated Values
To enhance usability, below is a table with pre-calculated values for the cardioid area using different parameters:
| a (units) | theta (degrees) | Area (square units) |
|---|---|---|
| 1 | 360 | 3.14 |
| 2 | 360 | 12.56 |
| 3 | 360 | 28.26 |
This table allows users to quickly reference common calculations, facilitating a smoother educational or professional workflow.
Example of Cardioid Area Calculator
Consider a cardioid with a parameter a = 2. Using the formula provided:
A = (1/2) * integral(2(1 + cos(theta)))^2 dtheta
The steps to solve this will be illustrated, including the integration process and calculation of the area, supplemented by a graphical representation to aid visual learners.
Most Common FAQs
A cardioid is a unique curve resembling the shape of a heart, commonly studied in polar coordinates.
From architectural design to acoustics, understanding the properties of cardioids can optimize various engineering and design tasks.
Yes, the calculator is versatile and can adapt to various units, making it a robust tool for international use.