A base of a trapezoid calculator simplifies the process of calculating one base's length when you know the trapezoid's area, the other base, and the height. This tool is invaluable in planning and designing projects where precise measurements are critical.
Formula of Base of a Trapezoid Calculator
To find the length of one base ("b one") of a trapezoid when the other base ("b two"), area ("A"), and height ("h") are known, you can use the following formula:
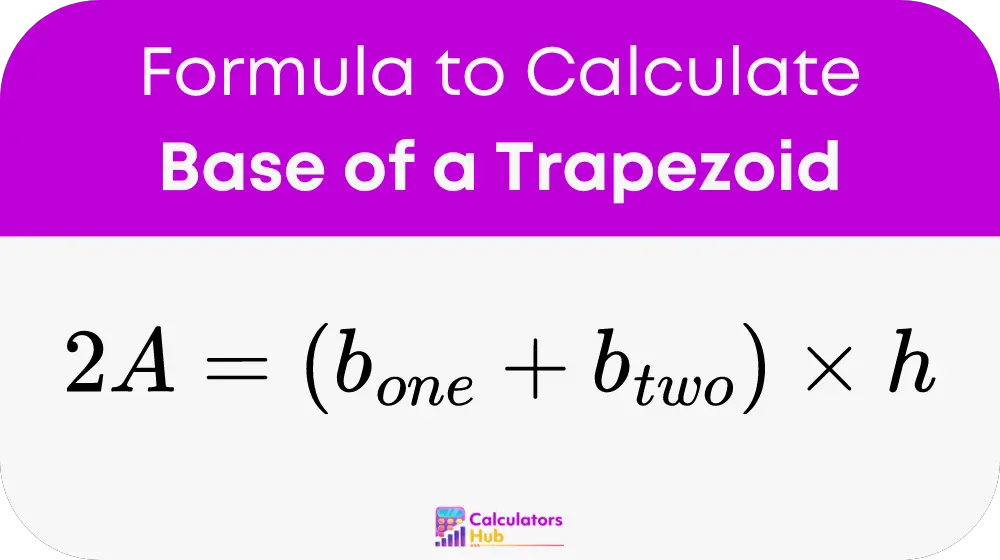
To isolate "b one":
- Multiply both sides by "2" to get: "2A = (b one + b two) * h"
- Divide both sides by "h" to find: "2A/h = b one + b two"
- Subtract "b two" from both sides to solve for "b one": "b one = 2A/h - b two"
This formula provides a straightforward method to compute the unknown base, ensuring accuracy and efficiency in your calculations.
Helpful Table
Below is a table with typical trapezoid measurements, providing quick reference values for "b one" based on given "A", "b two", and "h":
| Area (A) | Base Two (b two) | Height (h) | Base One (b one) |
|---|---|---|---|
| 100 sq units | 20 units | 10 units | 10 units |
| 150 sq units | 25 units | 15 units | 5 units |
| 200 sq units | 30 units | 20 units | 10 units |
This table helps users estimate or verify their calculations without performing complex operations.
Example of Base of a Trapezoid Calculator
Imagine designing a garden with a trapezoidal plot where one side (base two) measures 30 feet, the area is 600 square feet, and the height is 20 feet. To find the length of the opposite side (base one), use the formula:
"b one = 2*600/20 - 30 = 60 - 30 = 30 feet"
This calculation confirms that both bases of the plot are equal, simplifying the design process.
Most Common FAQs
A1: Yes, the calculator can compute either base as long as the area, the other base, and the height are known.
A2: Absolutely, it's a great tool for teaching and understanding trapezoid properties in a classroom setting.