This calculator simplifies finding the area enclosed between two curves. It automates the complex computations involved, enabling users to obtain accurate results efficiently, which is beneficial in both academic and professional environments.
Formula
The formula to calculate the area between two curves y = f(x) and y = g(x), for x = a to x = b where f(x) >= g(x) within the interval, is given by:
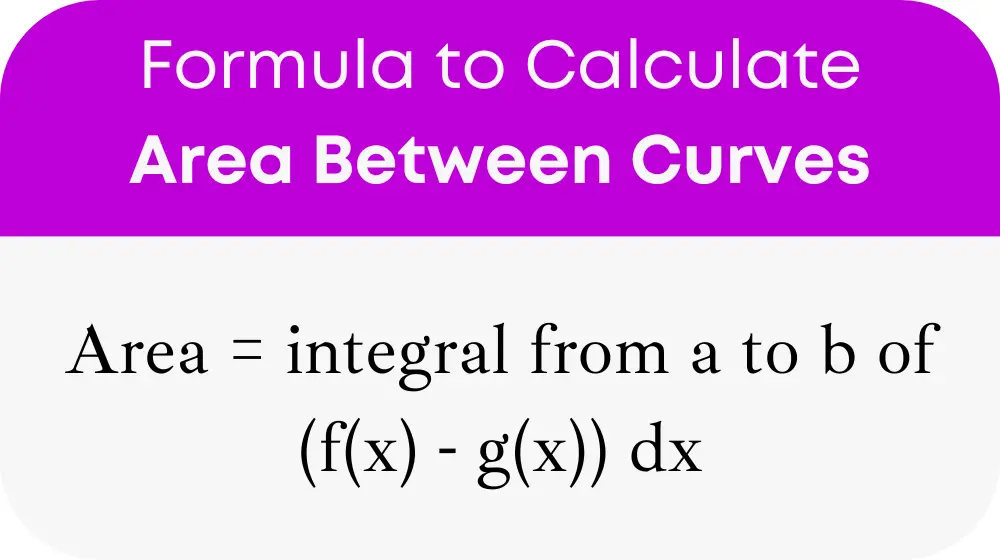
This formula uses integration to determine the precise area between the curves. Here, a and b are the bounds of integration, and dx signifies an infinitesimal change in x. Understanding each part of this formula is crucial for accurate calculations.
Table for General Terms and Useful Calculations
| Term | Definition |
|---|---|
| Integral (∫) | A mathematical symbol representing the integration process. |
| Bounds of Integration (a, b) | The limits between which the area under the curve is calculated, with 'a' and 'b' as limits. |
| dx | An infinitesimal change in x, indicating integration with respect to x. |
| f(x), g(x) | The equations of the curves between which the area is being calculated. |
| Useful Calculation | Description |
|---|---|
| Area between y = x^2 and y = 2x from 0 to 1 | Calculation involves integrating the difference (2x - x^2) from x = 0 to x = 1. |
| Area between y = sin(x) and y = cos(x) from π/4 to π/2 | Involves integrating the difference (cos(x) - sin(x)) from x = π/4 to x = π/2. |
Example
For instance, to calculate the area between the curves y = x^2 and y = x from x = 0 to x = 1, integrate the difference of the functions over the interval from 0 to 1. This example will show the step-by-step application of the formula, providing clear insight into its usage.
Most Common FAQs
Yes, the calculator can process any curves as long as the functions that define these curves are integrable over the specified interval.
The calculator's results are highly accurate, depending on the precision of the input functions and the numerical integration methods employed.
Indeed, for curves that intersect, the calculation involves identifying the intersection points to accurately define the integration bounds.