The Angle of Impact refers to the acute angle formed between the direction of a force and the plane it strikes. Calculators designed for determining the Angle of Impact are invaluable in fields requiring precise measurements of projectile trajectories, such as forensic ballistics and sports physics. By inputting the vertical and horizontal velocities of an object at the point of impact, the calculator provides the angle, thereby aiding in analysis and decision-making processes.
Formula of Angle of Impact Calculator
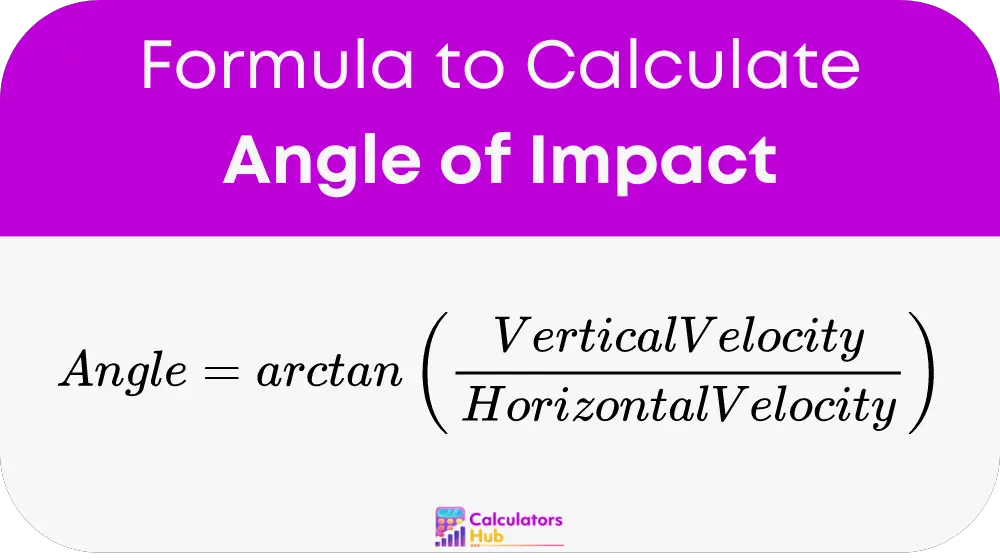
The calculation of the angle of impact is based on the formula:
Understanding the Formula Components
- arctan: This is the inverse tangent function, which calculates the angle whose tangent is the given ratio (vertical velocity divided by horizontal velocity).
- Vertical Velocity: This is the component of velocity vector pointing directly upwards from the surface of impact.
- Horizontal Velocity: This is the component of the velocity vector that is parallel to the impact surface.
Application Table
| Scenario | Vertical Velocity (m/s) | Horizontal Velocity (m/s) | Angle of Impact (degrees) |
|---|---|---|---|
| Basketball Free Throw | 5.9 | 4.5 | 52.4 |
| Soccer Ball Kick | 7.2 | 6.0 | 50.2 |
| Raindrop Falling | 9.0 | 0 | 90 |
This table helps visualize how different scenarios can be analyzed using the Angle of Impact Calculator.
Example of Angle of Impact Calculator
Scenario: A forensic scientist needs to determine the angle at which a bullet hit a wall, given that the bullet was traveling with a vertical velocity of 0 m/s and a horizontal velocity of 500 m/s.
Calculation:
angle of impact = arctan(0 / 500)
= arctan(0)
= 0 degrees
Interpretation: The bullet struck the wall at a 0 degree angle, i.e., it was perfectly horizontal at the point of impact.
Most Common FAQs
Vertical velocity is the component of an object's velocity directed vertically upwards as opposed to horizontal velocity. Which spreads parallel to the plane of impact. Its measurement is crucial in calculating the Angle of Impact.
Horizontal velocity affects the angle of impact by altering the tangent value in the arctan function of the formula. Higher horizontal velocities generally reduce the angle of impact if the vertical velocity is constant.
Yes, this calculator can be use for any object as long as you know vertical and horizontal velocities at the point of impact. It is ideal for analyzing projectile motions in sports and various types of collisions in forensic cases.