RPM stands for Revolutions Per Minute, a unit used to measure the frequency of rotation. It indicates how many full rotations a rotational body makes around a fixed axis in one minute. On the other hand, Radians per second (Rad/s) measures the angular velocity, or how quickly an angle is changing over time. Converting RPM to Rad/s is essential in various technical fields, particularly in designing and analyzing motors and mechanical systems where angular velocities need to be precisely controlled and understood.
Formula of RPM to Rad/s Calculator
The conversion from RPM to Rad/s is straightforward yet crucial. The formula used is:\
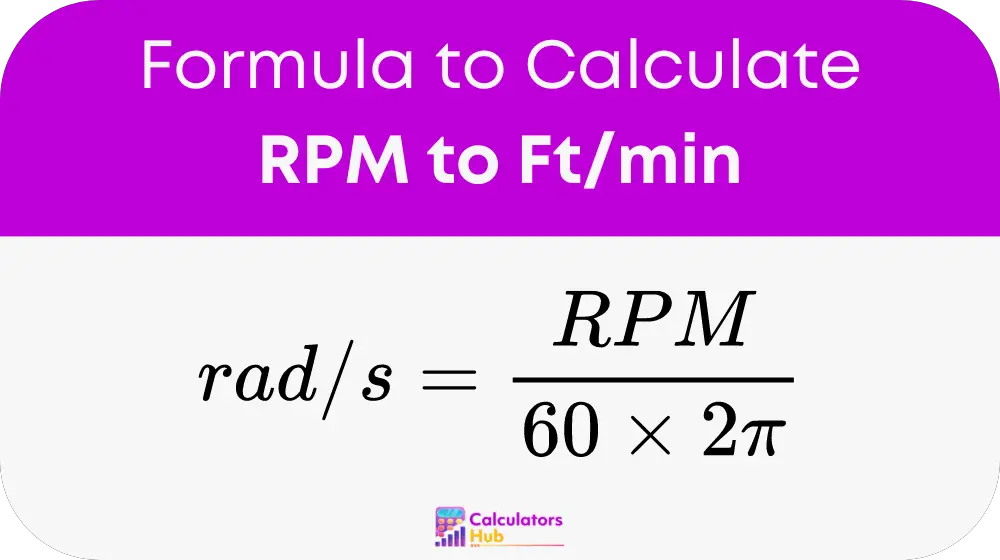
This formula converts RPM, a count of rotations per minute, into Rad/s, which is the angular velocity. By dividing RPM by 60, we convert it into revolutions per second since each minute contains 60 seconds. Multiplying by 2π2π (approximately 6.283) converts each revolution into radians (since one full revolution equals 2π2π radians).
Conversion Table
For practical applications, here’s a quick reference table that converts some common RPM values into Rad/s:
| RPM (Revolutions Per Minute) | Rad/s (Radians per second) |
|---|---|
| 60 | 6.283 |
| 120 | 12.566 |
| 600 | 62.832 |
| 1000 | 104.720 |
| 1500 | 157.080 |
Example of RPM to Rad/s Calculator
Consider a motor running at 1500 RPM, and you need to find its angular velocity in Rad/s. Using the formula:
Rad/s = 1500 / 60 x 2π = 157.08 Rad/s
This calculation shows that the motor’s angular velocity is approximately 157.08 Rad/s.
Most Common FAQs
Answer: This conversion is crucial for accurately describing and controlling the angular motion in mechanical systems, which is essential for system design and analysis.
Answer: Yes, this conversion universally applies to any rotational systems, regardless of the type of motor or mechanism.
Answer: Ensure accurate and precise input values. Understanding the formula and its components can also help in minimizing calculation errors.