An I-Beam Calculator is a practical tool used by engineers and construction professionals to estimate the maximum allowable load that an I-beam can support. This is essential for ensuring that structures are safe and capable of handling the intended loads with adequate safety margins.
Formula
The calculator uses a fundamental engineering formula:
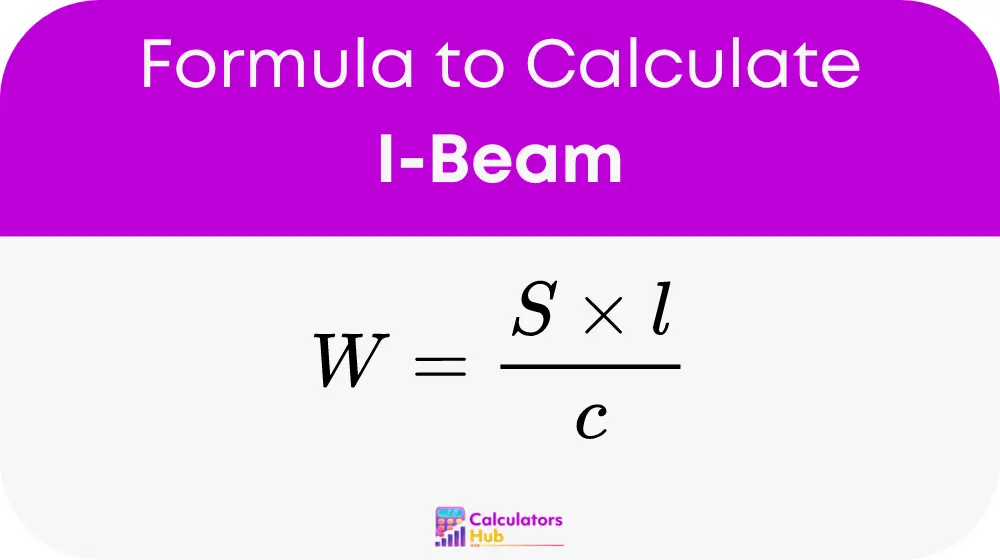
Where:
- W is the Maximum allowable uniformly distributed load (UDL),
- S is the Allowable stress for the material of the beam,
- I is the Moment of inertia of the beam’s cross-sectional area,
- c is the Maximum allowable deflection of the beam.
This formula helps in calculating the maximum load a beam can support, which ensures structures are both safe and efficient in using materials.
Table of Common Calculations
For ease of use and to facilitate quick checks, here is a table with common calculations for standard I-beam sizes and materials:
| Material | Beam Size | Allowable Stress (S) | Moment of Inertia (I) | Max Deflection (c) | Max Load (W) |
|---|---|---|---|---|---|
| Steel | 8×10 | 36,000 psi | 30 in^4 | 0.25 in | 4,320 lbs |
| Aluminum | 8×10 | 25,000 psi | 30 in^4 | 0.38 in | 1,973 lbs |
These values provide a quick reference and help users apply the calculator effectively without detailed manual calculations.
Example
Let’s consider a scenario where you need to calculate the load capacity for a standard steel I-beam:
- Input Values:
- Material: Steel
- Beam Size: 8×10
- Allowable Stress (S): 36,000 psi
- Moment of Inertia (I): 30 in^4
- Maximum Deflection (c): 0.25 in
- Calculation:
- Using the formula: W = (S x I) / c
- Substitute the values: W = (36000 x 30) / 0.25 = 4,320 lbs
- Interpretation:
- The beam can safely support a maximum uniformly distributed load of 4,320 lbs without exceeding its stress capacity or allowable deflection.
Most Common FAQs
A1: Steel is the most common material due to its high strength-to-weight ratio and excellent structural properties.
A2: The longer the beam, the greater the deflection under the same load. Hence, maximum load capacity decreases as beam length increases.
A3: Yes, the calculator can estimate the weight based on the material density and the volume derived from the beam’s dimensions.