The Effective Section Modulus Calculator is a structural engineering tool used to determine the bending resistance of thin-walled or slender cross-sections in steel and other materials. Unlike the regular section modulus, this calculator adjusts the values based on the effective width method, which accounts for local buckling in compression zones of thin elements.
This adjustment is critical in structural design because thin elements can buckle before yielding, reducing the actual strength of the section. Engineers use this tool when working with cold-formed steel, aluminum sections, or other lightweight structural members where local buckling is a concern.
This calculator belongs to the category of Structural Engineering and Mechanics Calculators.
formula of Effective Section Modulus Calculator
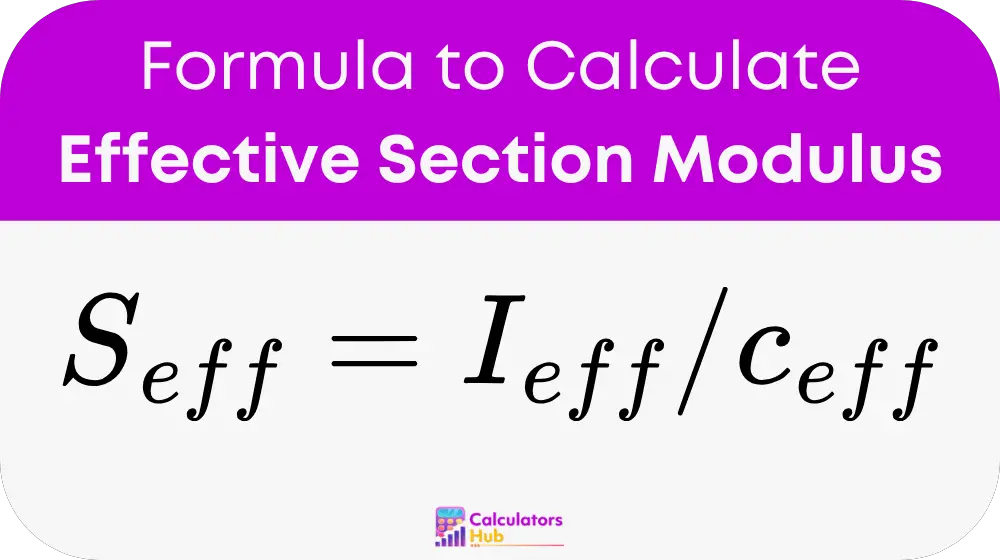
Where:
- S_eff = Effective Section Modulus (units: in³ or mm³)
- I_eff = Effective second moment of area, recalculated using only the effective width (b_eff) of compression elements (units: in⁴ or mm⁴)
- c_eff = Distance from the neutral axis to the outermost point of the effective section (units: in or mm)
Notes:
- Effective width (b_eff) is computed for each plate element using slenderness ratio (b/t) and design codes such as AISI or Eurocode.
- I_eff is derived by replacing the actual width with b_eff in the moment of inertia calculation.
- c_eff may shift if the effective section alters the position of the neutral axis.
General Terms Table for Quick Reference
| Term | Description | Relevance in Design |
|---|---|---|
| S_eff | Effective Section Modulus | Reflects true bending strength in thin-walled members |
| I_eff | Effective moment of inertia | Adjusted for local buckling using effective width |
| c_eff | Distance from neutral axis to extreme fiber in the effective section | Key for calculating bending stresses |
| b/t | Slenderness ratio of element (width/thickness) | Used to determine if local buckling adjustments are needed |
| b_eff | Effective width of element | Used in place of full width when recalculating I_eff |
Example of Effective Section Modulus Calculator
Let’s say we have a cold-formed steel C-section with the following properties:
- Full flange width (b) = 60 mm
- Thickness (t) = 1.2 mm
- Moment of inertia using full width (I) = 2.5 × 10⁴ mm⁴
- Distance from the neutral axis to the outermost fiber (c) = 40 mm
- Based on the code, the effective width (b_eff) for the flange is 40 mm
First, we recalculate the effective moment of inertia:
I_eff = (40 / 60) × 2.5 × 10⁴
I_eff ≈ 1.67 × 10⁴ mm⁴
Now, calculate the effective section modulus:
S_eff = 1.67 × 10⁴ / 40
S_eff ≈ 417.5 mm³
This shows that the usable bending capacity is significantly reduce due to the effect of local buckling.
Most Common FAQs
It gives a more realistic estimate of bending strength in thin-walled sections by adjusting for local buckling.
Use it when dealing with cold-formed steel, aluminum, or any slender elements prone to local buckling under compression.
It’s calculated using design codes (e.g., AISI, Eurocode) and depends on the slenderness ratio (b/t), loading, and support conditions.