The Bending Stress Calculator is a tool used in engineering and structural analysis to determine the bending stress within a material subjected to a bending moment. Bending stress is a critical factor in the design and analysis of structural elements such as beams, bridges, and other load-bearing components. It helps engineers assess whether a material can withstand the applied loads without failing or deforming excessively.
By using the Bending Stress Calculator, engineers can quickly and accurately calculate the stress distribution across the cross-section of a beam or similar structure. This ensures that the design is safe, efficient, and meets the required specifications. Understanding and calculating bending stress is essential for ensuring structural integrity and longevity.
Formula of Bending Stress Calculator
The formula used to calculate bending stress is:
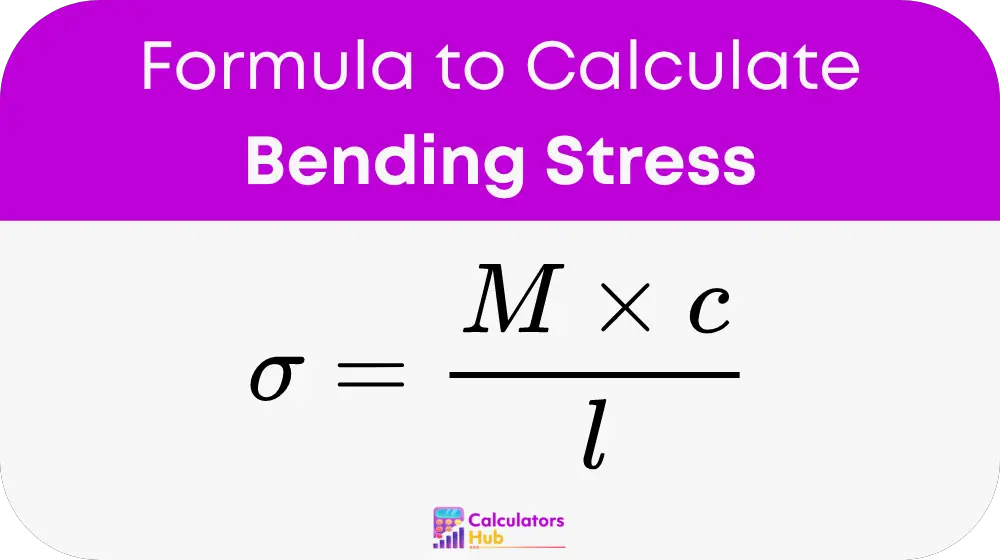
Where:
- σ is the bending stress, measured in pascals (Pa) or pounds per square inch (psi).
- M is the bending moment, measured in newton-meters (Nm) or pound-feet (lbf·ft).
- c is the distance from the neutral axis to the outermost fiber, measured in meters (m) or inches (in).
- I is the moment of inertia of the cross-section about the neutral axis, measured in meters to the fourth power (m^4) or inches to the fourth power (in^4).
This formula calculates the stress at the outermost fibers of the cross-section, where the material experiences the maximum stress due to bending. Accurate calculation of bending stress is crucial for determining the strength and durability of structural elements.
Useful Conversion Table
Below is a table that provides common terms and values related to bending stress calculations. This table can help users quickly understand the inputs required for the Bending Stress Calculator and make necessary conversions.
| Term | Description | Common Values |
|---|---|---|
| Bending Moment (M) | The moment applied to the structure causing it to bend. | 500 Nm, 1000 Nm, 1500 Nm; 300 lbf·ft, 600 lbf·ft, 900 lbf·ft |
| Distance from Neutral Axis (c) | The distance from the neutral axis to the outermost fiber of the section. | 0.1 m, 0.2 m, 0.3 m; 4 in, 8 in, 12 in |
| Moment of Inertia (I) | The geometric property of the cross-section that indicates its resistance to bending. | 1e-6 m^4, 2e-6 m^4, 3e-6 m^4; 1e-3 in^4, 2e-3 in^4, 3e-3 in^4 |
| Bending Stress (σ) | The calculated stress within the material due to the bending moment. | Varies based on inputs |
This table provides a quick reference for common values used in bending stress calculations, making it easier for users to input data and interpret the results effectively.
Example of Bending Stress Calculator
Let’s consider an example where you need to calculate the bending stress in a beam with the following characteristics:
- Bending Moment (M): 1000 Nm
- Distance from Neutral Axis (c): 0.2 m
- Moment of Inertia (I): 2e-6 m^4
Calculation
Using the formula:
σ = (M * c) / I
Substitute the values:
σ = (1000 Nm * 0.2 m) / 2e-6 m^4 σ = 200 Nm / 2e-6 m^4 σ = 100,000,000 Pa
Interpretation
The bending stress in the beam is 100 MPa (megapascals). This stress value can be compared with the material’s yield strength to ensure that the beam can safely carry the load without failure.
Most Common FAQs
Calculating bending stress is crucial because it helps engineers determine whether a structural element can withstand the applied loads without failing. Bending stress analysis ensures that the design is safe, efficient, and meets the necessary strength requirements. Failure to accurately calculate bending stress can lead to structural failures, which can have severe consequences in construction and manufacturing.
To reduce bending stress in a beam. You can increase the moment of inertia (I) by selecting a cross-section with a larger area or a shape that offers greater resistance to bending. Additionally, reducing the applied bending moment (M) or increasing the distance from the neutral axis (c) can also help lower the bending stress.
Yes, the Bending Stress Calculator can be used for various materials. As long as the correct values for the bending moment (M). Distance from the neutral axis (c), and moment of inertia (I) are provide. This allows the calculator to provide accurate stress values for a wide rdange of materials. From steel to concrete to wood.