The Beam Reinforcement Ratio Calculator is a crucial tool used in structural engineering to determine the reinforcement ratio of a concrete beam. The reinforcement ratio is a measure of the amount of steel reinforcement used in a concrete beam relative to its cross-sectional area. This ratio is vital for ensuring that the beam can handle the expected loads safely and efficiently without failing. A proper reinforcement ratio helps in optimizing the design, balancing cost, safety, and structural performance.
This calculator assists engineers and architects in designing beams that meet specific strength requirements. By calculating the reinforcement ratio, professionals can verify whether the beam design adheres to the structural codes and standards, ensuring the safety and durability of the structure.
Formula of Beam Reinforcement Ratio Calculator
The formula to calculate the Beam Reinforcement Ratio (ρ) is:
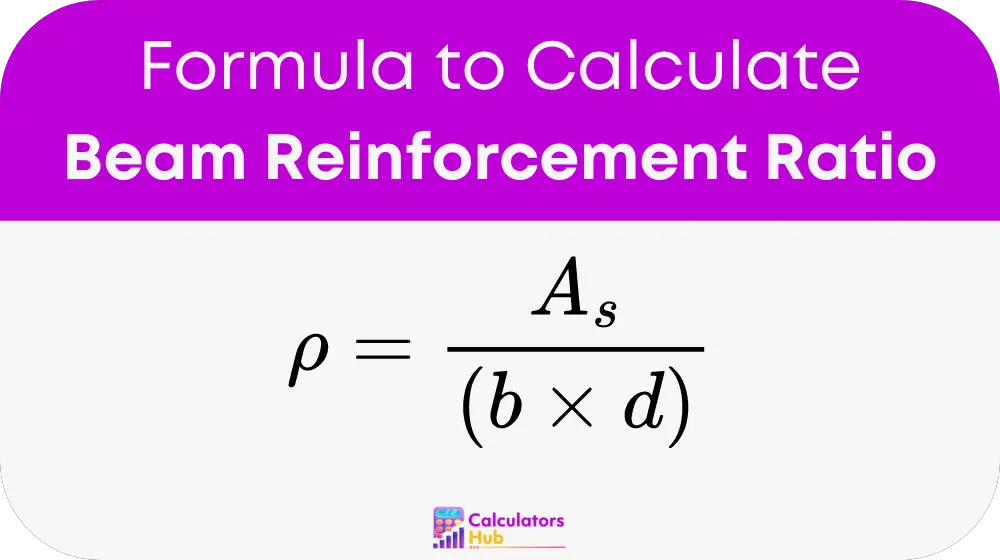
Where:
- ρ is the reinforcement ratio, a dimensionless quantity.
- A_s is the area of steel reinforcement (in square units, such as mm² or in²).
- b is the width of the beam (in the same units as d).
- d is the effective depth of the beam, which is the distance from the extreme compression fiber to the centroid of the tensile reinforcement (in the same units as b).
Steps to Calculate the Reinforcement Ratio
- Determine the Area of Steel Reinforcement (A_s):
Calculate the total area of all steel reinforcement bars used in the beam. This is typically provided in the design specifications and is measured in square units (e.g., mm², in²). - Measure the Width of the Beam (b):
Measure the width of the concrete beam. Ensure that the measurement is in the same units as used for the effective depth. - Determine the Effective Depth of the Beam (d):
The effective depth is the distance from the extreme compression fiber to the centroid of the tensile reinforcement. This measurement is also in the same units as the beam width.
By inputting these values into the formula, you can easily calculate the reinforcement ratio, helping you to ensure that the beam design is both safe and efficient.
General Terms and Reference Table
Here’s a table with common reinforcement ratios used in practice. This reference can help engineers quickly assess whether their calculated reinforcement ratio is within typical ranges for various beam types and conditions.
| Reinforcement Ratio (ρ) | Description | Application |
|---|---|---|
| 0.002 - 0.005 | Minimum reinforcement ratio | Common in lightly loaded beams, ensuring minimum safety. |
| 0.005 - 0.010 | Typical reinforcement ratio | Standard for most residential and commercial beams. |
| 0.010 - 0.015 | High reinforcement ratio | Used in heavily loaded beams or special structural elements. |
| > 0.015 | Very high reinforcement ratio, usually not recommended unless specially designed | Requires detailed analysis for safety and cost. |
Example of Beam Reinforcement Ratio Calculator
Let’s work through an example to demonstrate how to calculate the reinforcement ratio.
Suppose you have the following data for a beam:
- Area of Steel Reinforcement (A_s): 1200 mm²
- Width of the Beam (b): 300 mm
- Effective Depth of the Beam (d): 500 mm
Using the formula:
Reinforcement Ratio (ρ) = (A_s) / (b * d)
ρ = 1200 / (300 * 500) = 1200 / 150000 = 0.008
In this example, the reinforcement ratio is 0.008. This value falls within the typical reinforcement range, making the beam suitable for most standard applications.
Most Common FAQs
The reinforcement ratio is critical because it determines how much steel is used relative to the concrete in the beam. A proper ratio ensures that the beam can safely carry the expected loads, balancing strength and material cost.
If the reinforcement ratio is too high, the beam may be over-reinforced, leading to unnecessary costs and potential issues with ductility. If it’s too low, the beam may not have enough strength, increasing the risk of failure under load.
An acceptable reinforcement ratio typically falls within a standard range, as outlined in structural codes. Comparing your calculated ratio with industry standards or consulting with a structural engineer can help ensure it’s appropriate for your specific application.