This calculator measures the axial deformation, or the change in length, of an object when a specified force is applied along its length. The results from this calculator are vital for engineers to ensure that the materials and designs used in construction and manufacturing can withstand expected loads without excessive deformation that could lead to structural failure.
Formula of Axial Deformation Calculator
The formula to calculate axial deformation is a fundamental expression derive from Hooke’s Law, which describes how the strain (deformation) of a material is directly proportional to the applied stress:
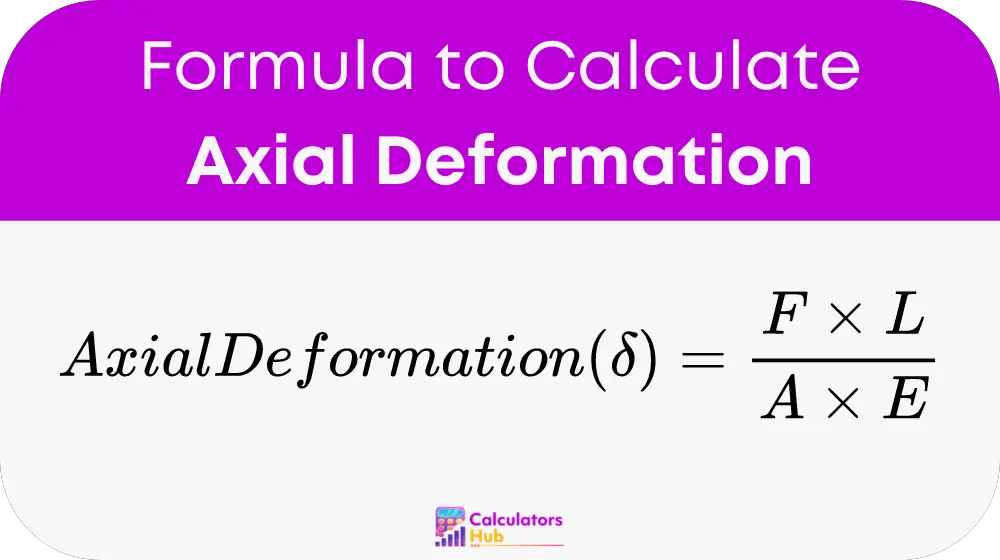
Where:
- Axial Deformation (δ): The change in length due to the applied force, measured in units like millimeters or inches.
- Force (F): The axial force applied to the object, generally measured in newtons or pounds.
- Length (L): The original length of the object before any force is apply, measured in meters or feet.
- Area (A): The cross-sectional area of the object, measured in square meters or square inches.
- Modulus of Elasticity (E): Also known as the elastic modulus, this is a measure of a material’s ability to deform elastically (i.e., non-permanently) when a force is apply. It is measured in pascals or psi (pounds per square inch).
Table for General Terms
To enhance understanding, here’s a table defining key terms related to the Axial Deformation Calculator:
| Term | Definition |
|---|---|
| Axial Deformation (δ) | The change in length of an object under axial force |
| Force (F) | The axial force applied |
| Length (L) | Original length of the object |
| Area (A) | Cross-sectional area through which the force is applied |
| Modulus of Elasticity (E) | Material property describing stiffness |
Example of Axial Deformation Calculator
Consider a steel rod with a length of 2 meters, a cross-sectional area of 0.01 square meters, and a modulus of elasticity of 200 gigapascals. If a force of 10,000 newtons is apply to this rod, the axial deformation would be calculate as follows:
Axial Deformation (δ) = (10,000 N * 2 m) / (0.01 m² * 200 GPa) = 0.01 meters or 10 millimeters
This example shows that the steel rod would elongate by 10 millimeters when subjected to the given load, helping engineers assess whether this deformation is acceptable for their specific application.
Most Common FAQs
A1: Temperature can significantly impact the modulus of elasticity and thus the axial deformation. Most materials expand with increasing temperature, which can reduce their stiffness and increase deformation under the same loads.
A2: If the material remains within its elastic limit, axial deformation is generally reversible. However, if the deformation has surpassed the elastic limit, leading to plastic deformation, it becomes permanent.
A3: Calculating axial deformation is crucial for ensuring that structures can withstand their intended loads. Without excessive deformation that might compromise their integrity or function.