The Aluminum Heat Expansion Calculator is a valuable tool used in various industries to predict the change in length of aluminum objects due to temperature fluctuations. This article aims to provide a comprehensive overview of the calculator, its formula, practical applications, and frequently asked questions.
Formula of Aluminum Heat Expansion Calculator
The formula used in the Aluminum Heat Expansion Calculator is:
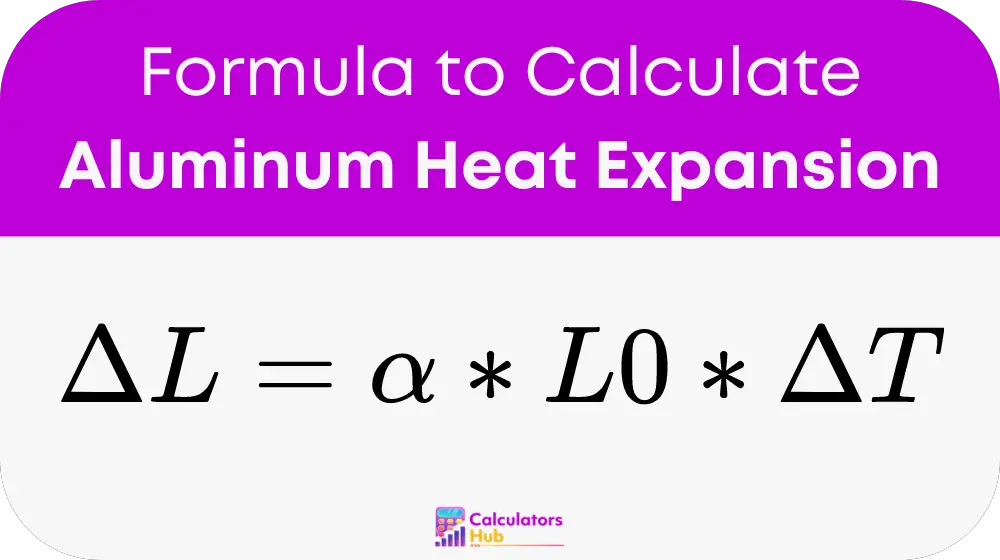
Where:
- ΔL is the change in length (in meters or any other unit of length)
- α is the coefficient of linear expansion for aluminum (in per degree Celsius or per Kelvin)
- L0 is the original length of the aluminum object (in meters or any other unit of length)
- ΔT is the change in temperature (in degrees Celsius or Kelvin)
The coefficient of linear expansion for aluminum is approximately 22.2 x 10^-6 per degree Celsius or per Kelvin.
Commonly Searched Terms Table
| Term | Description |
|---|---|
| Aluminum Heat Expansion | Explains the phenomenon of aluminum expanding or contracting due to temperature changes |
| Linear Expansion Calculator | Tool used to calculate the change in length of materials, including aluminum, with temperature changes |
Example of Aluminum Heat Expansion Calculator
Let’s consider an example to illustrate how the Aluminum Heat Expansion Calculator works:
Suppose we have an aluminum rod with an original length (L0) of 1 meter. If the temperature increases by 50 degrees Celsius (ΔT), we can calculate the change in length (ΔL) using the formula:
ΔL = α * L0 * ΔT
Given that the coefficient of linear expansion for aluminum (α) is 22.2 x 10^-6 per degree Celsius, we can plug in the values:
ΔL = (22.2 x 10^-6) * 1 * 50
ΔL = 0.00111 meters
So, the aluminum rod will expand by approximately 0.00111 meters when the temperature increases by 50 degrees Celsius.
Most Common FAQs
A: When aluminum is heated, its particles gain energy and vibrate more, causing the material to expand. Conversely, when aluminum cools, its particles lose energy and contract, resulting in a decrease in length.
A: While the formula provided is specific to aluminum, similar principles apply to other materials. However, each material has its own coefficient of linear expansion, so separate calculations would be necessary.