A Divergence Angle Calculator helps measure the spread of a beam of light, laser, or any other radiative energy as it moves away from its source. This calculation is crucial in optics, laser engineering, astronomy, and other scientific applications where 光束发散角 affects performance and precision.
Divergence angle plays an essential role in determining beam quality, focusing ability, and efficiency in various industries, including telecommunications, medical imaging, and manufacturing. A correct calculation ensures accurate beam alignment and helps in optimizing optical systems for improved results.
Formula for Divergence Angle Calculator
The divergence angle can be calculated using the following formula:
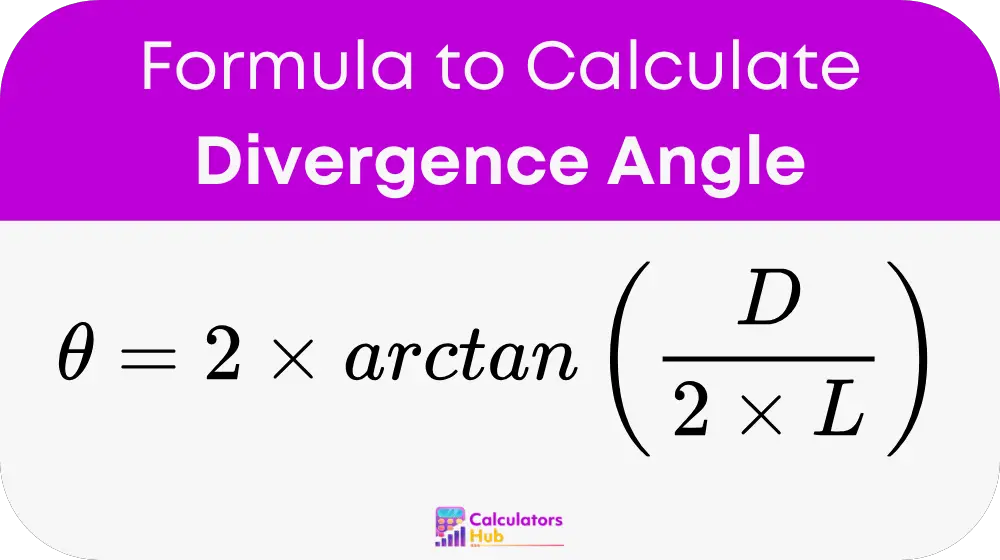
地点:
- θ (Theta): Divergence angle (in radians or degrees).
- D: Beam diameter at a given distance (meters or feet).
- L: Distance from the source to the measurement point (meters or feet).
This formula provides an accurate way to determine the beam divergence based on measurable parameters. Knowing the divergence angle is 键 for applications requiring high precision, such as laser cutting, satellite communications, and fiber optics.
Commonly Used Terms and Predefined Calculations
Below is a reference table with commonly used terms and estimated divergence angles for different beam types:
| 梁类型 | Approximate Divergence Angle (Degrees) |
|---|---|
| 激光笔 | 0.1 - 2 |
| LED灯 | 5 - 60 |
| 手电筒光束 | 10 - 45 |
| 光纤 | 2 - 30 |
| Telescope Laser | 0.5 - 3 |
| 投影仪光束 | 10 - 25 |
The actual divergence angle can vary depending on the optics used and the beam characteristics. These values serve as general reference points for estimation.
Example of Divergence Angle Calculator
Suppose you have a laser beam with a diameter of 5 mm 距离 2米 from the source. Using the formula:
Divergence Angle (\u03B8) = 2 \u00d7 arctan(5 / (2 \u00d7 2000))Converting the values into meters:
Divergence Angle (\u03B8) = 2 \u00d7 arctan(0.005 / 4)使用科学计算器:
\u03B8 \u2248 2 \u00d7 arctan(0.00125) \u2248 0.143 degreesThis means the laser beam has a divergence angle of approximately 0.143度, which is consider very narrow, making it suitable for high-precision applications like optical communication and laser targeting.
最常见的常见问题解答
Calculating the divergence angle is crucial for ensuring the accuracy and efficiency of optical and laser systems. It helps optimize focusing, beam alignment, and energy distribution.
Reducing the divergence angle can be achieve by using high-quality collimating optics, such as lenses or mirrors, and by improving beam quality through better optical components and alignment techniques.
For laser communication systems, a low divergence angle (below 1 degree) is generally prefer to ensure minimal signal loss and high transmission accuracy over long distances.