The Empirical Probability Calculator is a tool used to calculate the probability of an event occurring based on observed data or experimental results. Unlike theoretical probability, which is based on expected outcomes derived from assumptions and mathématique models, empirical probability is calculated from actual experimental data. This makes it particularly useful in fields such as statistics, science, economics, and decision-making, where real-world observations are crucial to making predictions.
The calculator helps users determine the likelihood of a particular outcome based on the ratio of favorable results to total trials. By inputting the number of favorable outcomes and total trials into the formula, individuals can assess how likely an event is to occur, given observed trends.
Formula of Empirical Probability Calculator
The formula for calculating empirical probability is:
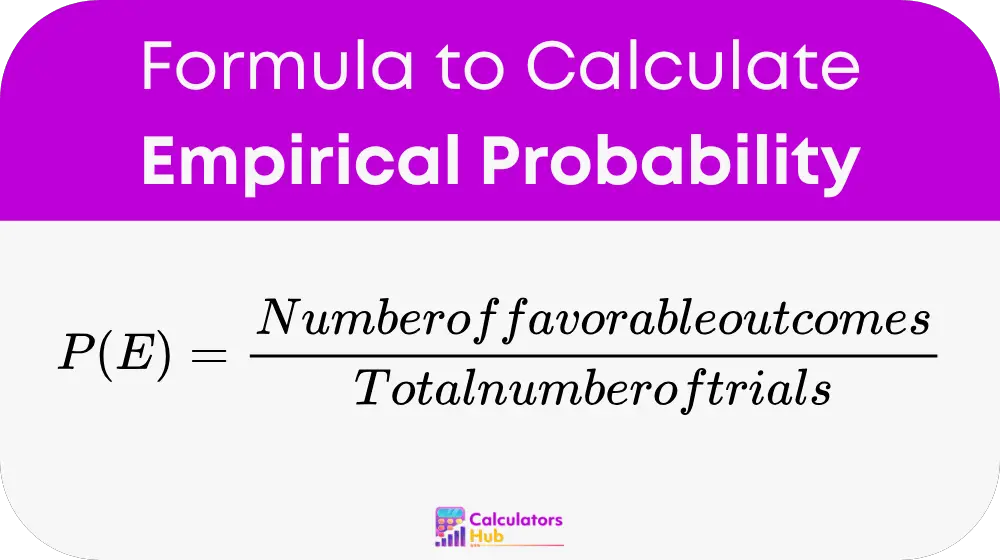
Où :
- P(E) is the empirical probability of event E occurring.
- Number of favorable outcomes is the number of times event E occurs during the trials.
- Total number of trials is the total number of experiments or trials conducted.
This formula calculates the ratio of successful outcomes (or favorable results) to the total number of experiments or trials, providing an estimate of the probability of the event based on real-world data.
Common Search Terms and Helpful Conversion Table
Here’s a table with common terms and definitions related to empirical probability that people may search for when using the calculator. This table helps clarify clé concepts and ensures users fully understand the calculation process:
| Long | Description |
|---|---|
| Probabilité empirique | The probability of an event based on observed data rather than theoretical predictions. |
| Résultats favorables | The outcomes in which the event of interest occurs. |
| Essais | The total number of experiments or observations conducted. |
| Nombre total d'essais | The sum of all experiments or observations performed in the study. |
| Probabilité expérimentale | Another term for empirical probability, used interchangeably. |
| Événement | A specific outcome or group of outcomes that is being studied in a probability experiment. |
This table serves as a reference for users to understand the terms and concepts when using the Empirical Probability Calculator.
Example of Empirical Probability Calculator
Let’s go through a practical example to see how the Empirical Probability Calculator works.
Scénario:
You roll a fair die 100 times and want to calculate the probability of rolling a “4.”
- Number of favorable outcomes (rolling a “4”) = 18
- Total number of trials (rolls) = 100
En utilisant la formule :
P(E) = (Number of favorable outcomes) / (Total number of trials)
P(E) = 18 / 100 = 0.18
In this case, the empirical probability of rolling a “4” based on 100 trials is 0.18 or 18%.
This empirical probability is based on observed data, which may differ from the theoretical probability of rolling a “4” (which would be 1/6 or approximately 0.167). The empirical probability reflects the observed frequency of the event occurring in the trials conducted.
FAQ les plus courantes
Empirical probability is the probability of an event occurring based on observed data rather than theoretical models. It is calculated by dividing the number of favorable outcomes by the total number of trials conducted.
To calculate empirical probability, use the formula: P(E) = (Number of favorable outcomes) / (Total number of trials). Count the number of times the event occurs (favorable outcomes) and divide it by the total number of trials to get the empirical probability.
Empirical probability is based on actual observed data from experiments or trials, whereas theoretical probability is based on mathematical models and assumptions about how events should behave. Empirical probability may differ from theoretical probability due to variability in real-world experiments.