A Desaceleración Calculadora de distancia ayuda drivers, engineers, and physicists determine the distance an object travels while slowing down before coming to a stop. This calculation is critical in road safety, vehicle design, aerospace engineering, and physics experiments, where accurately predicting stopping distances is essential for preventing accidents and improving braking systems.
Deceleration distance depends on the inicial velocidad, final speed, and rate of deceleration. Whether analyzing car braking distances, train stopping points, or aircraft landing rollouts, this calculator provides valuable insights into how much distance is required to safely bring a moving object to a halt.
Formula for Deceleration Distance Calculator
Los Deceleration Distance (d) is calculated using the following equation derived from kinematic motion principles:
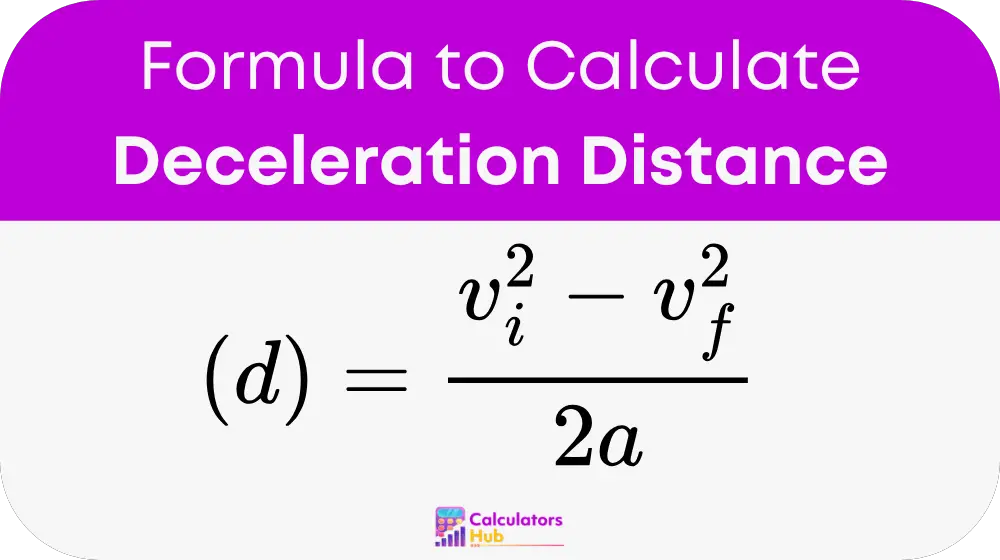
Lugar:
d = Distance traveled during deceleration (m)
v_i = Velocidad inicial (m/s)
v_f = Final velocity (m/s)
a = Deceleration (m/s²) (must be a positivo valor)
This formula ensures that even if the deceleration rate is high, the stopping distance remains accurate. A higher initial velocity or lower deceleration rate resultará en un longer stopping distance.
Deceleration Distance Reference Table
La siguiente tabla proporciona una estimación deceleration distances based on different initial speeds and deceleration rates, assuming the object comes to a complete stop (v_f = 0).
| Velocidad inicial (m/s) | Deceleration (m/s²) | Distancia de parada (m) | Common Application |
|---|---|---|---|
| 10 | 5 | 10 | Bicycle braking |
| 20 | 7 | 28.57 | Car stopping at city speed |
| 30 | 8 | 56.25 | Car stopping on highway |
| 50 | 10 | 125 | High-speed train braking |
| 70 | 12 | 204.17 | Aterrizaje de aviones |
This table allows drivers, engineers, and safety analysts to estimate stopping distances quickly without manual calculations.
Example of Deceleration Distance Calculator
A car is traveling at 25 m / s and comes to a complete stop with a deceleration of 5 m/s².
Step 1: Apply the Deceleration Distance Formula
d = (25² - 0²) ÷ (2 × 5)
Step 2: Compute the Result
d = (625) ÷ (10)
re = diez metros
Esto significa que el car requires 62.5 meters to stop completely bajo estas condiciones.
Preguntas frecuentes más comunes
Deceleration distance depends on initial speed, braking force, friction, and road conditions. A higher initial speed or lower deceleration rate leads to a longer stopping distance, while strong braking force and good traction help reduce it.
Understanding stopping distances helps drivers, engineers, and policymakers design safer roads, improve vehicle braking systems, and establish appropriate speed limits. It is especially important in preventing collisions and improving emergency braking strategies.
Yes, deceleration distance is critical in aviation. Ayuda a determinar la pista de largo needed for safe landings, accounting for aircraft speed, braking efficiency, and environmental conditions like wind resistance and tire friction.